How to Conceptually Teach Solving Equations

Please Excuse My Dreadful Acrostic Sentence is more like it, but in all fairness, it does help students remember the order of operations.  And hopefully you have spent a bit of time teaching them why.

Come wintertime, kindergarten teachers across the north struggle to help their children to get their snow clothes on.  And it doesn’t matter how many times they teach or model it, there is always the kid who has their boots on and is struggling to put on their snow pants, or the kid with gloves on trying to zip up their coat begging their teacher for help because they just can’t get it.  Many frustrated kindergarten teachers post the steps with pictures to help remind students and reduce their neediness.

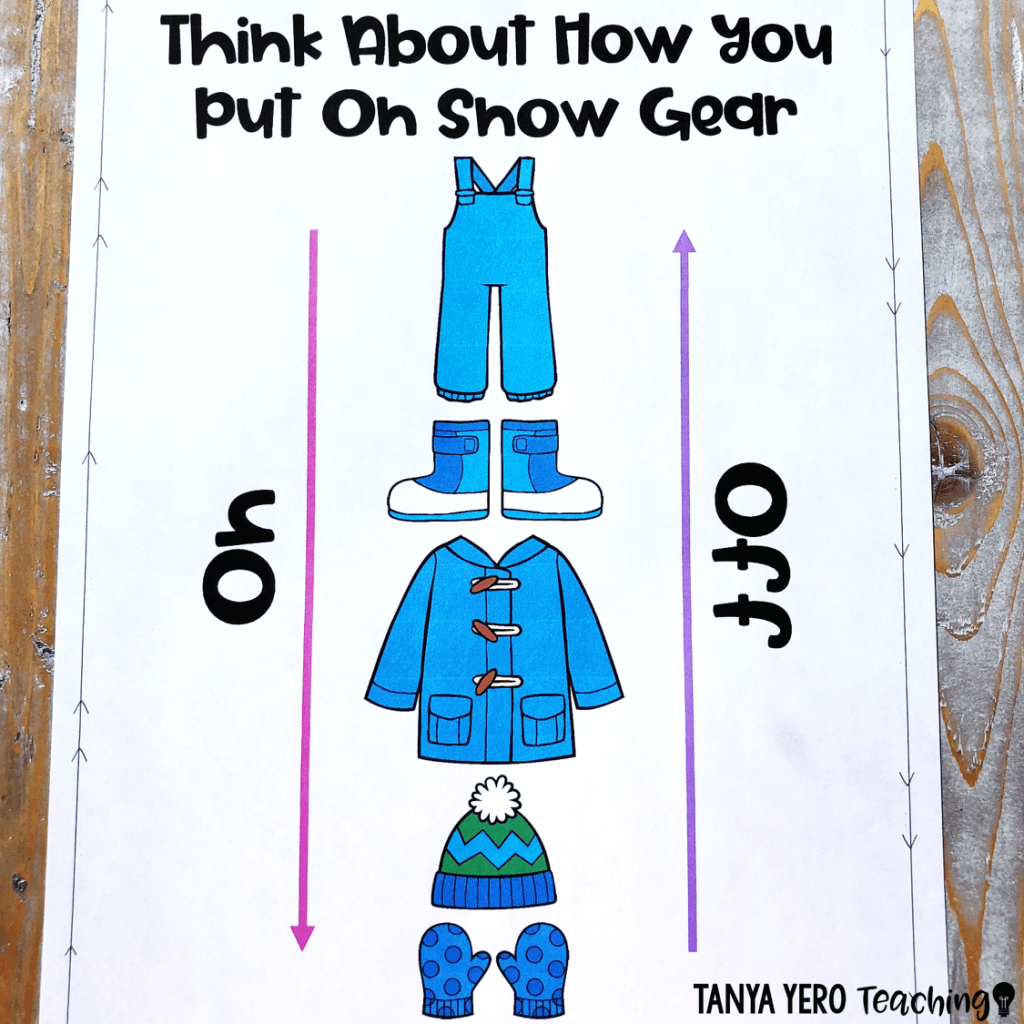
Just as there is a way to do things, so also there is a way to undo them.  You unzip zippers; you untie laces; you take off hats.  Not only do you undo all of the snow items, you also do them in a certain order.  Mittens come off before unzipping your coat.  Boots come off before snow pants.  In fact, kids find that the order they do them in is the exact opposite of how they went on.

In learning to solve equations, students sometimes are so used to the order of operations, that they forget that they need to work backwards to “undo” or “take off” each part.  They will see the equation 2x + 7 = 13, and try to divide first (usually just the 2 and 13) and then add.   Other students will try multiplying 13 by 2 and then subtracting 7.  Even others will do some combination of these.  When students are directed back to the getting dressed in snow clothes process, however, we review how we have to work backwards to get to the variable, which is what we want.  One strategy for helping students understand how to work backwards is to help them use what they already know.  In the equation 2x + 7 =13, have them put numbers on top of the operations indicating the order of operations such as below:

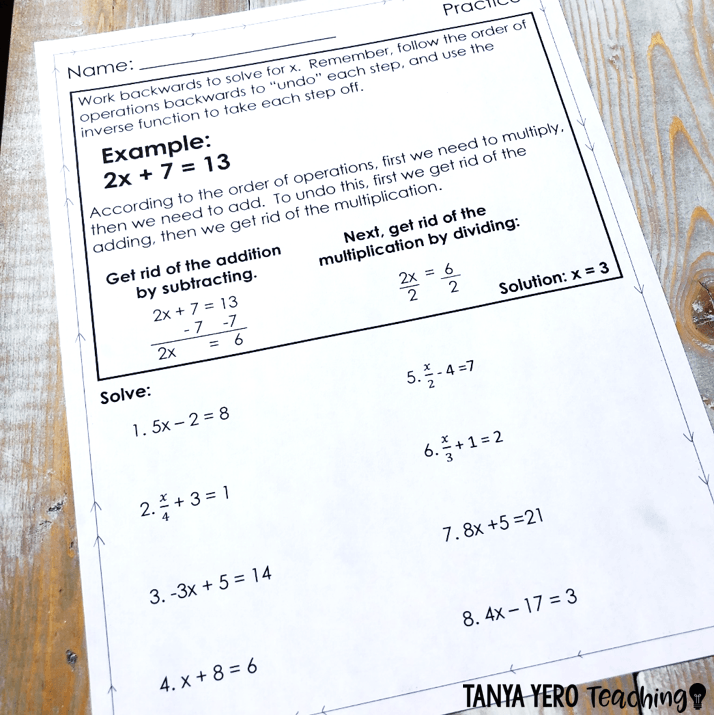
Ask your students: If these are the two steps that build this equation, what is the first thing that we want to take off? Why do we do this?  How do we do this?  Students should be able to reference the snow clothes analogy that we take the last thing off, because we are working backwards, and we take it off by “undoing it”.  In this case, they may say that we take off the + 7, because it was the last thing put on and we are working backwards to get to the x.  We do this by undoing the subtraction by adding 7 to both sides.  You can repeat these questions with any remaining steps.

If your students need more examples or live in a warmer climate, you can introduce other ordered procedures common for their daily life:

• To get a piece of paper out of their folder, they have to first unzip the backpack, then they have to open the folder.  To pack to leave, they have to close their folder, and then zip up their backpack. 
• To pick up students, the bus stops, the red lights go on, and the doors open.  To leave, the doors close, the red lights go off, and the bus starts moving again.

By realizing that solving equations is simply undoing the order of operations, students can clearly and articulately explain how to solve even more complex equations, such as 3(x + 4) – 5 = 10, which is just a fancy way of saying that x = 1.  This also paves the way for them to progress towards more complex math in the years to come.

Your kids have been preparing to do this math since kindergarten and they will succeed if they understand the “why”  behind the math.

Looking for printables for your students to practice this skill? Sign up below to our newsletter to receive your free printables!