How To Teach Decimals Conceptually

Decimals are a huge part of your math curriculum. They are connected to so many standards in various domains; place value, fractions, and measurement. Teaching this topic conceptually will give your students the exposure and confidence they need to see beyond standard algorithms and truly understand the reasoning behind their work. It’s easy to teach the algorithms for adding, subtracting, multiplying, and dividing decimals and breeze through this content, but you’re missing out on an opportunity to bring concrete understanding to these four operations. 

Here is a breakdown of how I teach the four operations in connected to decimals in my classroom.

Addition and Subtraction of Decimals:

Adding decimals – the easiest of the four, addition of decimals seems to be a nice starting point for students. Before I start on addition I really want my students to have a strong conceptual understanding of decimal place values, how they relate to one another (the hundredths place is one-tenth the value of the tenths place, but 10 times greater than the thousandths place), and be able to visually represent decimals using models. 

We use place value disks and a place value chart to represent the decimals we are adding. Students will regroup disks when needed and see how that act changes the value of their numbers. 

We also practice representing addition and subtraction of decimals with hundredth grids. Students will use different colored highlighters to represent the values being combined and cross out values when subtracting. 

Multiplication of Decimals:

I think multiplication and division of decimals are far more “fun” than addition and subtraction. There are various ways to model and represent decimals using pattern blocks, fraction tiles, hundredth grids, and base ten blocks. 

Here are some examples of the types of questions we review in class.

We work on these visual representations long before I introduce any algorithms. With a concrete understanding of multiplication with decimals your students will be able to understand the work behind the formula. 

Division of Decimals:

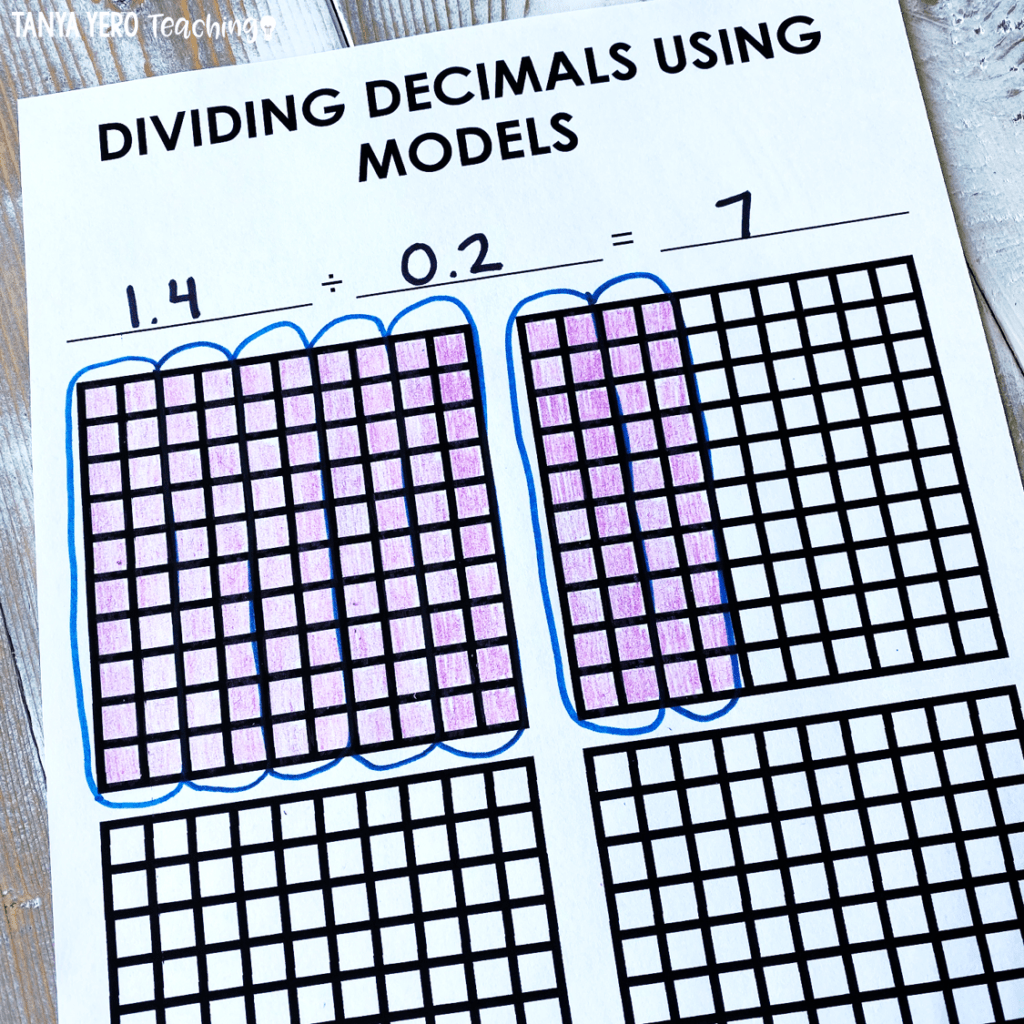
You want to continue modeling and requiring students to conceptually understand this operation, just like the other three. Again, we use models to represent dividing a decimal by a decimal, decimal by a whole number, and a whole number by a decimal. Your students should be able to represent each of these scenarios using a hundredth grid. I integrate word problems anywhere I can so students have practice identifying which operation to use. 

If you set your students up with conceptual understanding of decimals, this will help with fraction and measurement standards later on in the year. Next year’s teacher will also appreciate your efforts and you’re building number sense too!

Sign up below to receive our newsletter and gain access to your templates so you can start teaching decimals conceptually TODAY!