Open-ended tasks are a great way to promote productive struggle. I use this type of questioning to drive my math instruction. Designed to have several correct answers, they empower your students to select their own pathways to solutions.
A strong understanding of the curriculum and the standards you are expected to teach is a must for writing conceptual based questions. Standards are multifaceted. Some are deceiving. It’s like looking at abstract art. If you’re just looking at the surface, you’re missing the layers of depth underneath. You also need to identify which standards parallel one another because you can use that to your advantage. Here are 4 tips to use when selecting questions and tasks for your students or when creating questions to promote productive struggle.
1.) Requiring students to work backwards
This a great strategy that brings depth and conversation to any math class. This sort of questioning often produces multiple strategies for solving problems.
2.) Wording
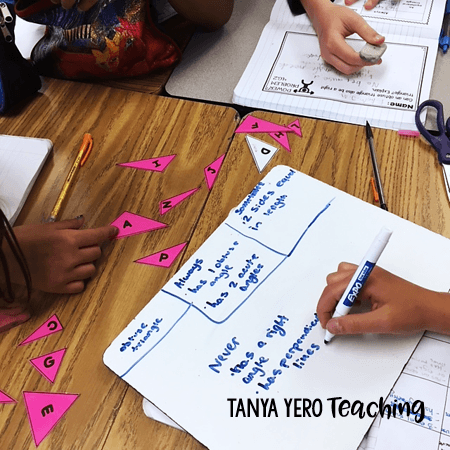
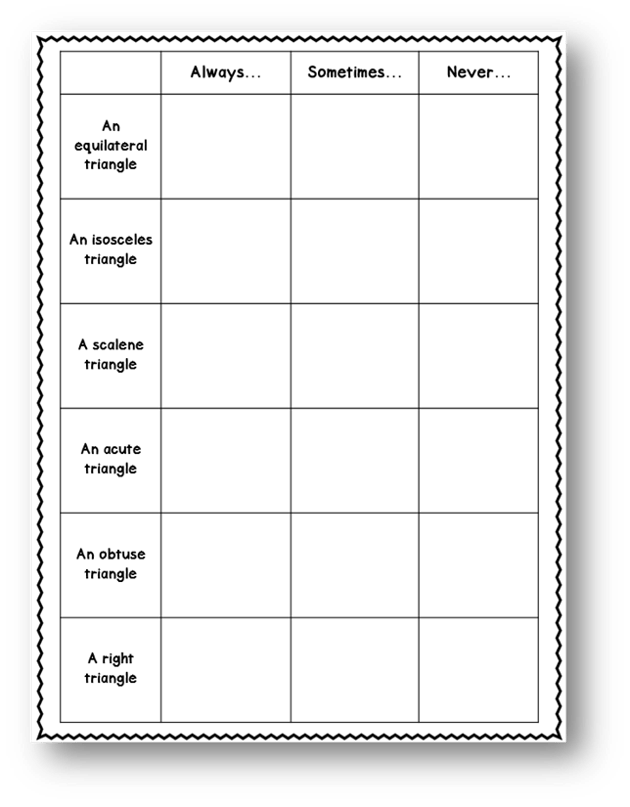
Exclude wording that may provide too much assistance or give students too much information. The triangle activity pictured below was extremely vague. I didn’t specify exactly what I was looking for in each box, nor did I explain what the headers at the top meant. I gave each group a bag of triangles and asked them to complete the first row about equilateral triangles. I wanted to see how far my students could carry themselves first.
3.) Resolving conflict within group discussions
When I was reviewing place value earlier this past school year, I gave my students the following task: “Create a number that has the digit 2 in the place value that is 1/10 less than the 2 in the number 3.628. Next, put a 7 in the hundreds place.” Then, I had each student write his or her answer on the whiteboard. Next, I asked each group to go through the list of answers and agree or disagree with each number. There was a lot of disagreement and justification from groups.
4.) Visual representation
Students providing answers by using diagrams, symbols, models, and/or words – Most students operate procedurally. When tasked with multiplying 2/3 by ¾, they can easily rely on background knowledge to provide the answer. But could they create models showing the operation? Push your students to create visual representations of their work.
Integrating these exercises into your math curriculum will help you create productive struggle. Productive struggle will lead to questioning, discussion, and justification which are the backbone of conceptual understanding.
My line of Power Problems are designed to target conceptual understanding of standards thru productive struggle. They are available for grades 3rd-6th.